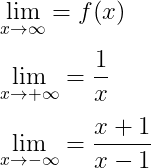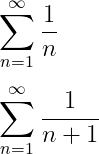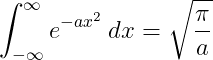The infinity symbol is widely used in mathematics to represent a value that has no limit.
In LaTeX, you can display this symbol using the \infty command. This ensures proper formatting in mathematical equations.
\[ \left ( -\infty ,+\infty \right ) \]
\[ \left ( -\infty ,+\infty \right ) \]
Indeterminate Forms of Infinity in LaTeX
In mathematics, indeterminate forms involving infinity (∞) appear in various limit calculations. Below is a table showing different forms and their LaTeX representations:
| Command | Output |
|---|---|
1^{\infty} |
\(1^{\infty}\) |
\infty^{0} |
\(\infty^{0}\) |
\frac{\infty}{\infty} |
\(\cfrac{\infty}{\infty}\) |
0\cdot\infty |
\(0\cdot\infty\) |
\infty-\infty |
\(\infty-\infty\) |
When writing equations in LaTeX, it is important to use the correct format depending on whether the equation appears within text (inline mode) or on a separate line (display mode).
To insert equations inside a text line, use \(...\) or $...$.
Range of R $\left(-infty,0 \right]$
Output :
Range of R \((-\infty,0]\)
For equations that need to appear separately from text, use \[...\].
Range of R $\left(-infty,0 \right]$
\[ \lim_{x \to \infty}f(x) \]
Output :
Range of R (-∞,0]
Use of Infinity Symbols in Mathematics
The infinity symbol (∞) is frequently used in mathematics for various purposes, such as defining domains, limits, summations, and integrals. Below are some key applications of the ∞ symbol in LaTeX.
Defining Domain and Range
The infinity symbol is commonly used to define the domain and range of a function. In LaTeX, this can be written using the \infty command.
\[ R \in (-\infty,a]\cup[b,+\infty) \]
Output :
And if you look at the code above, you can see that the \in command has been used for the ∈ symbol.
Limits Involving Infinity
In calculus, the ∞ symbol is widely used in limit expressions, often with a positive +∞ or negative -∞ sign.
\[ \lim_{x\to\infty}=f(x) \]
\[ \lim_{x\to+\infty}=\frac{1}{x} \]
\[ \lim_{x\to-\infty}=\frac{x+1}{x-1} \]
Output :
Summation Series
The summation symbol ∑ is often used with ∞ to denote an infinite series.
\[ \sum_{n=1}^{\infty} \frac{1}{n} \]
\[ \sum_{n=1}^{\infty} \frac{1}{n+1} \]
Output :
Infinity in Integration
In integrals, the ∞ symbol is used to represent integration over an ∞ range, which is common in quantum physics and probability theory.
\[ \int_{-\infty}^{\infty}e^{-ax^{2}}\:dx=\sqrt{\frac{\pi}{a}} \]
Output :